The Galton Board: Randomness and the Gaussian Curve

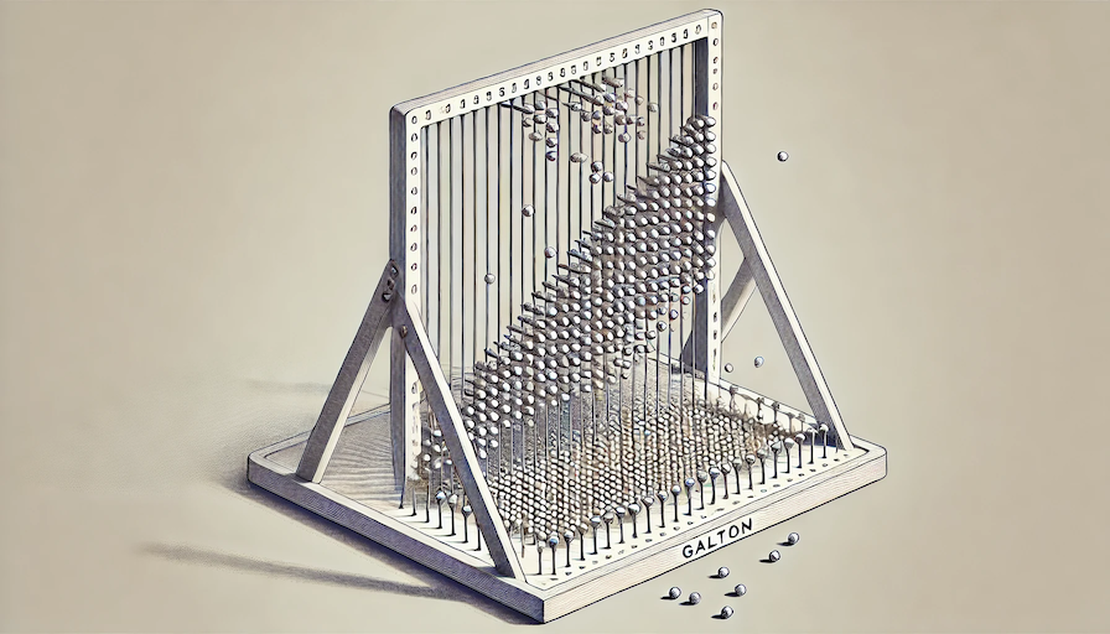
The Galton Board, also known as the quincunx, is a simple yet powerful device that demonstrates randomness and the beauty of probability. Invented by Sir Francis Galton, to demonstrate the Central limit theorem, this board visually illustrates how individual random events can combine to form a predictable pattern: the Gaussian, or normal, distribution.

What Is a Galton Board?

At its core, the Galton Board is a vertical frame with rows of pegs. When a bead is dropped from the top, it encounters the pegs, each of which deflects it randomly left or right. By the time the bead reaches the bottom, it lands in one of many bins. Drop thousands of beads, and you’ll notice something remarkable: the distribution of beads across the bins forms a bell curve.

The Gaussian Curve in Action

The bell curve, or Gaussian curve, represents the normal distribution—a fundamental concept in statistics. It’s fascinating that randomness at the micro level (each bead’s path) creates order at the macro level (the shape of the curve).

This phenomenon happens because the randomness of the pegs follows the principles of probability. Each bead’s path is an example of the Central Limit Theorem, which explains why independent, random events often result in a normal distribution when aggregated.

Real-World Applications

The Galton Board isn’t just a curiosity; it has practical implications. Many natural phenomena, from heights of people to measurement errors, follow a normal distribution. The board provides an intuitive way to understand why these patterns occur.

In fields like finance, biology, and physics, the principles behind the Galton Board help us model uncertainty, variability, and randomness. Even algorithms in machine learning often rely on Gaussian distributions to make predictions and analyze data.

Why It Matters

The Galton Board is more than a historical artifact; it’s a gateway to understanding randomness and probability. It reveals how chaos can lead to order and offers insights into the behavior of systems influenced by countless small, independent variables.

Conclusion

The simplicity of the Galton Board hides a profound truth about the natural world. From randomness comes predictability, and from unpredictability arises the elegant symmetry of the Gaussian curve. It’s a reminder that even in chaos, patterns emerge—if only we look closely enough.

⚠️ Please take a look at the LLM Disclaimer